Ky Fan inequality is a fascinating result in mathematics, specifically within the realm of matrix theory and inequalities. It provides a powerful tool for comparing the eigenvalues of two Hermitian matrices and has wide-ranging applications in diverse fields. This article delves deep into the intricacies of Ky Fan inequality, exploring its proof, significance, and various applications.
What is Ky Fan Inequality?
Ky Fan inequality establishes a relationship between the eigenvalues of the sum of two Hermitian matrices and the sums of their respective eigenvalues. More formally, let A and B be two n x n Hermitian matrices with eigenvalues λ₁(A) ≥ λ₂(A) ≥ … ≥ λₙ(A) and λ₁(B) ≥ λ₂(B) ≥ … ≥ λₙ(B), respectively. Let C = A + B, with eigenvalues λ₁(C) ≥ λ₂(C) ≥ … ≥ λₙ(C). Then, Ky Fan inequality states:
∑ᵢ₌₁ᵏ λᵢ(A + B) ≤ ∑ᵢ₌₁ᵏ [λᵢ(A) + λᵢ(B)] for k = 1, 2, …, n.
This inequality essentially states that the sum of the k largest eigenvalues of the sum of two Hermitian matrices is less than or equal to the sum of the k largest eigenvalues of each individual matrix added together.
Proof of Ky Fan Inequality
The proof of Ky Fan inequality utilizes the variational characterization of eigenvalues, also known as the min-max theorem. This theorem provides a way to express the eigenvalues of a Hermitian matrix in terms of the Rayleigh quotient. The detailed proof involves constructing specific subspaces and applying the min-max theorem iteratively. While mathematically involved, it elegantly demonstrates the relationship captured by the inequality.
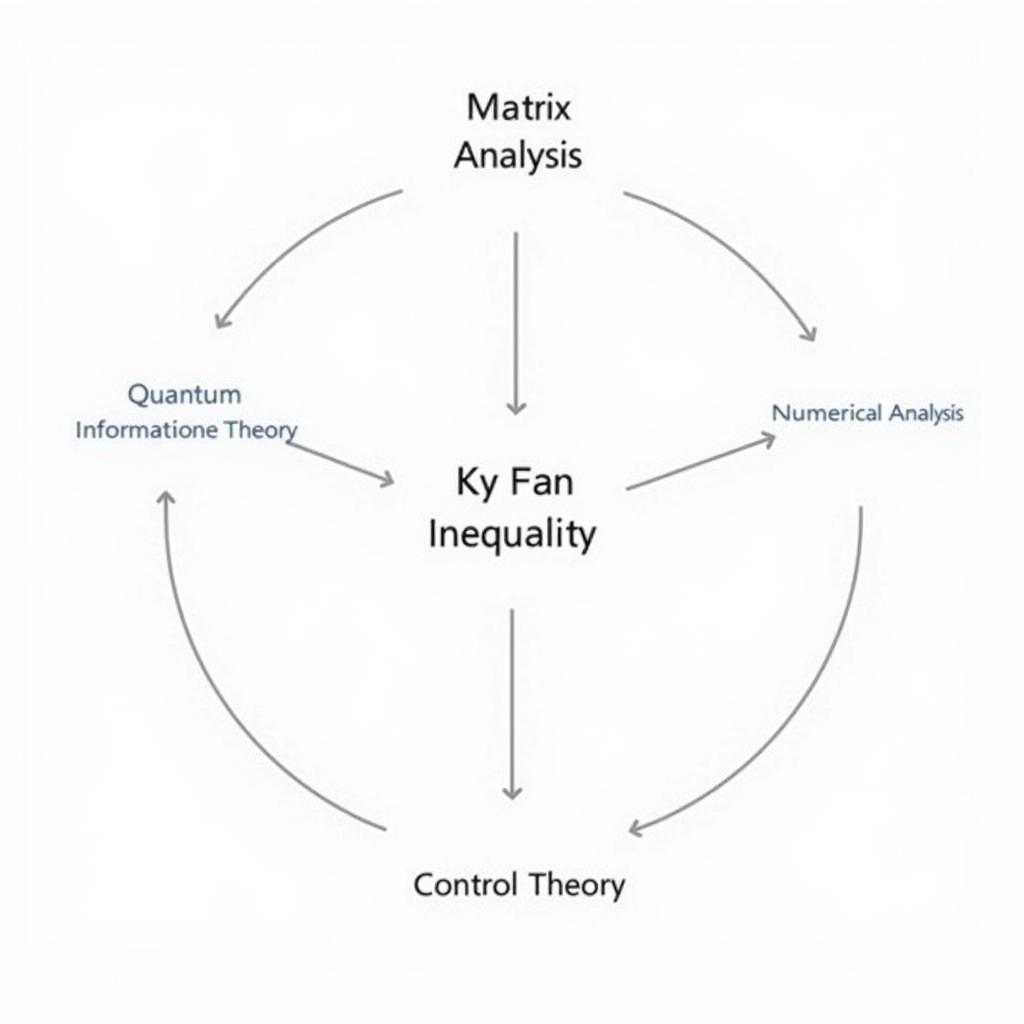
Applications of Ky Fan Inequality
Ky Fan inequality finds applications in various fields, including:
- Matrix Analysis: It’s a fundamental tool for understanding the behavior of eigenvalues under matrix addition and plays a crucial role in other matrix inequalities.
- Quantum Information Theory: Ky Fan inequality is used in the study of entanglement measures and quantum channel capacities.
- Numerical Analysis: It can be utilized to analyze the convergence of certain numerical algorithms involving matrices.
- Control Theory: The inequality has applications in the design and analysis of control systems, particularly in the context of robust control.
Why is Ky Fan Inequality Important?
Ky Fan inequality is more than just a mathematical curiosity; it provides crucial insights into the interplay of eigenvalues and matrix operations. Its importance stems from its wide applicability and its ability to provide bounds on eigenvalues, which are essential in many practical scenarios.
 Ky Fan Inequality Applications
Ky Fan Inequality Applications
Conclusion
Ky Fan inequality stands as a significant result in matrix theory, offering a powerful tool for understanding and manipulating eigenvalues. Its elegance lies in its concise formulation and far-reaching applications across diverse fields. By providing a robust relationship between the eigenvalues of Hermitian matrices, it continues to be a valuable asset in both theoretical and practical applications.
FAQ
- What are Hermitian matrices?
- What are eigenvalues?
- How is the min-max theorem used in the proof?
- What is a specific example of Ky Fan Inequality in action?
- What are some other related matrix inequalities?
- How does Ky Fan inequality relate to other matrix norms?
- What are the limitations of Ky Fan Inequality?
Need support? Contact us at Phone Number: 0903426737, Email: fansbongda@gmail.com Or visit us at: Lot 9, Area 6, Gieng Day Ward, Ha Long City, Gieng Day, Ha Long, Quang Ninh, Vietnam. We have a 24/7 customer support team.


